Mathematical and Historical Background to
"All That Glitters...: A Review of Psychological
Research on the Aesthetics of the Golden Section"

Christopher D. Green

Department of Psychology
York University
North York, Ontario M3J 1P3
CANADA

christo@yorku.ca

Author's Note

This is the mathematical and historical background to the article entitled "All that glitters...," published in Perception (1995). This material was in the original draft of the paper, but much of it was ultimately cut from the published version. After reading it, the article continues at Section 2 of the published version.

The golden section was among the very first topics of scientific psychological research, Fechner first fixed his analytical gaze upon it as early as the 1860s. Since that time it has been the focus of sporadic research programs, winning the attention of structuralists, gestaltists, behaviorists, social psychologists, psychiatrists, and neuroscientists at various points in time. Needless to say, all of the attention it has received has not been favorable, and there have been enough cranks involved with golden section research to sully its once-golden reputation.

This review is primarily focused on the reputed aesthetic properties of the golden section, both the primary historical import and the leading program of research associated with it. There have been other research programs associated with the golden section, however, including its relation to the timings of human cortical evoked potentials (Schafer, 1974); the structure of ethical cognition (Lefebvre, 1985); the cognitions of sufferers of anxiety, depression, and agoraphobia (Schwartz & Garamoni, 1989; Schwartz & Michelson, 1987); the body images of phantom limb sufferers (Fischer, 1969); consumers' perceptions of products and of retail environments (Crowley, 1991a, 1991b; Crowley & Williams, 1991); and even the proportion of wins of championship sports franchises (Benjafield, 1987).

The most productive non-aesthetic research program that will not be discussed in any detail in this review, however, has been that concerned with interpersonal relations led by Adams-Webber, Benjafield, and a group of psychologists at Brock University in Ontario, Canada. (See Adams-Webber, 1977a, 1977b, 1978, 1979, 1980, 1985; Adams-Webber & Benjafield, 1973; Adams-Webber & Davidson, 1979; Adams-Webber & Rodney, 1983; Benjafield, 1983, 1984, 1985; Benjafield & Adams-Webber, 1976; Benjafield & Green, 1978; Benjafield & Pomeroy, 1978; Kahgee, Pomeroy, & Miller, 1982; Lee & Adams Webber, 1987; Pomeroy, Benjafield, Rowntree, & Kuiack, 1981; Romany & Adams-Webber, 1981. See also their critics Marczewska 1983; Messick 1987; Rigdon & Epting, 1982; Shalit, 1980; Stradling, Tuohy, & Harper, 1990; Tuohy, 1987; Tuohy & Stradling, 1987; Walton 1982; Webley, 1984, 1985.)

Nevertheless, this review is restricted to an aesthetic focus. It is intended to fulfil the needs of both historical and scientific researchers. There are several different reasons one might be interested in a review of golden section research. One very general reason is that thought concerning golden section has a long history, stretching back possibly to the ancient Egyptians. A review of research into phenomenon that has for so long captured the interest of humans from so many different cultures might be of interest in its own right. A second reason, focussed more specifically on the history of psychology, is that studies of the golden section were among the very first empirical studies conducted in psychology; they take their place alongside Weber's and Fechner's psychophysical studies, Ebbinghaus' memory studies, and Helmholtz's studies of tone and color perception. Since that time the golden section has captured the interest of many psychological researchers, including some of the most illustrious names in the discipline. A third reason -- one emphasizing the science of psychology -- is that the psychological phenomena associated with the golden section, despite repeated attempts to show them to be nonexistent or mere methodological artifacts, simply refuse to go away. Although many researchers have concluded that the effects are illusory, the more carefully conducted studies have fairly consistently shown that there is, in fact, a set of phenomena that require explanation, though no one has yet produced an explanation, both adequate and plausible, that has been able to stand the test of time. Consequently, I propose to review the pre-scientific history of the golden section, its later emergence as a topic of interest to early scientific psychologists, and the procedures and conclusions of contemporary psychologists.

The paper is structured as follows: Section 1 introduces the basic features of the golden section (1.1), presents its more interesting and relevant (to the rest of the review) mathematical properties (1.2), and occurrences of the golden section in nature that have led some to believe it constitutes a sort of natural law (1.3). Section 2 surveys Ancient (2.1), and Medieval and Renaissance (2.2) perspectives on the number. Section 3 reviews empirical research conducted on its reputed aesthetic properties between the mid-19th century and about 1960. Section 4 examines the more recent theories and findings of psychologists, focusing particularly on concerted efforts to show that golden section effects are nothing but artefacts, and the stubborn resilience of the phenomenon many have tried to make go away. Finally, a concluding section summarizing the findings of the review, constitutes a brief Section 5. To begin, however, I turn to the question of what, exactly, the golden section is. [NB: Only Sections 1 and 2 are included in this version. Those interested in the rest of the paper should continue from the end this Introduction to Section 2 of the published version of the paper.]

The golden section stands alongside of π and e as the most important irrational constants in mathematics. It is often symbolized by the Greek letter φ, and is approximately equal to 1.61803398875. The origins of its various names are matters of some dispute. Berlyne (1971) claims that the reference to gold, "was adopted in large measure, because of vague associations with the 'golden mean'" (p. 229). Kepler (1596), however, referred to the Pythagorean Theorem and φ as the mathematical equivalents of gold and precious gems as far back as the 16th century. Fowler (1982) claims that the first use of "goldner Schnitt" appears in Martin Ohm's Die Reine Elementar-Mathematik (1835), and that its first titular appearance was in A. Wiegang's Der Allgemeine Goldene Schnitt uns Sein Zusammenhang mit der Harminischen Theilung (1849). It was the publications of Adolf Zeising's Neue Lehre von den Proportionen des menschlischen Körpers (1854), Äesthetische Forschungen (1855), and Der goldne Schnitt (1884), however, that did the most to widely popularize the name. Again according to Fowler (1982), the first English language use to the term, "golden section", seem to have been in James Sully's article on aesthetics in the 1875 edition of Encyclopaedia Britannica. Sully credits Zeising with the formulation of the hypothesis that the most aesthetically pleasing division of a line is at the golden section, but the source of this belief seems actually to be much more ancient.

The symbol φ, on the other hand, derives from the initial letter of the name of the great Greek architect and sculptor, Phidias. Phidias was a proponent of the aesthetic qualities of the ratio, going so far as to incorporate them into the basic dimensions of his most famous work, the Parthenon (see, e.g., Ogden, 1937). Huntley (1970), however, says that the appellation, φ, was not adopted until "the early days of the present century" (p. 25). Fechner (1876) and Witmer (1894), for instance, used a circle with a dot in the center to symbolize the golden section.

Constants such as π, e, and φ gain their importance from being the solutions to basic mathematical problems. In addition, they perhaps gain their special mystique from being irrational numbers; their exact values never being known. Just as π is the ratio of the circumference of any circle to its diameter, and e is the solution to the expression lim(1+1/n)ⁿ, the solution to the equation x²=x+1, is another of these long-standing problems in mathematics. The exact solution to the problem is

1±√5.
2

The positive solution is φ -- approximately 1.618 -- and the negative solution is approximately -0.618. For convenience, I shall denote the negative solution as -φ', and its negative -- approximately 0.618 -- as φ'. A number of interesting relations hold between these numbers and various derivatives. To begin with, φ' is equal to both φ-1 and to 1/φ, or φ^-1. Thus, φ-φ' = φxφ' = 1. Just as φ^-1 is equal to φ-1, so φ² is equal to φ+1, or φ¹+φ⁰. Similarly, φ³ is equal to φ²+φ¹, and so on; φⁿ = φ^n-1+φ^n-2, for any value of n. A number of other interesting mathematical and geometrical properties are discussed below.

Claims about the ubiquity of the golden section in mathematics and nature have often been made. φ is the limit of the ratio two sequential numbers in any Fibonacci series; that is, any series of integers in which x_n=x_n-1+x_n-2. The best known example of such series is 1, 1, 2, 3, 5, 8, 13, 21, ..., in which the series is begun with two 1s. Notice that although the ratios of two adjacent numbers oscillate above and below φ, they also approximate it increasingly closely as the series progresses:

It is not necessary that a Fibonacci series begin with two 1s. Any two integers can be used to generate such a series, but the limit of the ratios of two adjacent numbers in the series is always φ. Consider the Fibonacci series beginning -2, 10, 8, 18, 26,44, 70, 114. The ratios of its adjacent members are:

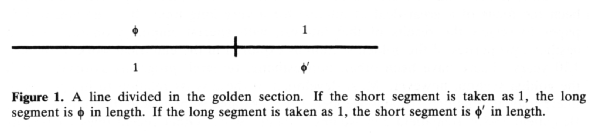
Geometrically, φ has many interesting properties as well. For instance, imagine that a line is divided into a ratio corresponding to φ' (i.e., that the line is broken into sections of approximately 62% and 38% of its overall length; see Figure 1). The ratio of the length of the longer segment to that of the shorter segment resulting from such a division is equal to φ. Moreover, the ratio of the line's overall length to that of the longer segment is also φ, and the ratio of its overall length to that the shorter segment is φ², or φ+1. It is because of these properties that φ is often referred to as the "golden section" of a line (although, technically, the number typically called by this name is actually φ').

Extending this knowledge to two dimensions, imagine that one of the segments of a line divided at the golden section were "folded" at a 90° angle to the other, and a rectangle was formed based on this L-shaped foundation, as in Figure 2a. This figure is called the "golden rectangle". The golden rectangle can be constructed quite simply. Starting with a square, swing an arc, centered on the midpoint of the bottom line segment of the square, from an upper corner of the square to a point collinear with the bottom segment. Build a rectangle on this base. (See Figure 2b.)

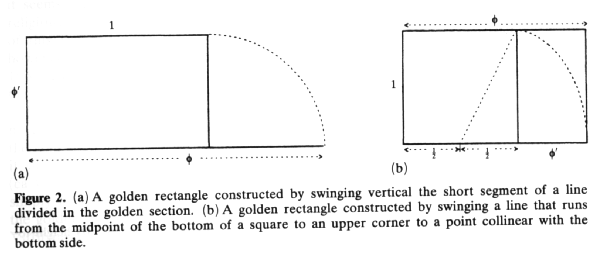
A special feature of the golden rectangle places it among a set of geometrically important rectangles as well. Imagine that a diagonal were drawn across a rectangle, and then another line were drawn from one of the "open" corners, perpendicular to the diagonal, and through to the opposite side, as in Figure 3. In rectangles in which the ratio of the long side to the short side corresponds to the square root of some number, x, the line perpendicular to the diagonal will cross the long side of the rectangle at a point corresponding to 1/x of its length. For instance, in a √2 rectangle (approx. 1.414:1), a line drawn from a corner, and perpendicular to the diagonal, divides the long side in half (Figure 3a). In a √3 rectangle (approx. 1.732:1), it sections off 1/3 of its length (Figure 3b). In a √4 rectangle (=2:1), it sections off 1/4 of its length (Figure 3c), etc.

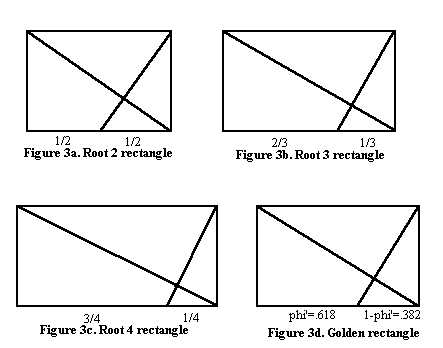
A similar construction in a golden rectangle sections off 1-φ' (=.382) of the length of the long side, and leaves a longer segment equal in length to that of the short side (Figure 3d). Further, if one were to drop a line perpendicular to the long side across the rectangle, to two interior quadrilaterals would correspond to a square and a golden rectangle. If a square were sectioned off from this new, smaller golden rectangle, the remaining section would itself be a golden rectangle as well. This process can be continued indefinitely, resulting in an indefinitely long series of smaller and smaller squares and golden rectangles (Figure 4). This figure is sometimes called "Whirling Squares". If one were to connect with a spiral curve the outermost points at which each golden rectangle meets its companion square, the resulting figure would correspond to Bernoulli's "logarithmic spiral", which is given in polar coordinates by ln(r)=θ (where ln is the natural logarithm, r is the radius, and θ is the angle).

Although not widely recognized as such until fairly recently, the whirling squares is an example of the infinitely deep self-similarity characteristic of fractals. With the recent explosion of popular interest in fractals (see, e.g., Dewdney, 1988, chaps. 1-2; Gleick, 1987, chap. 4; Lauwerier, 1987/1991), interest in the golden section has been rekindled with a new view to its relation to fractals (see e.g., Kappraff, 1991).

Extending the process to the third dimension, one can construct the "golden solid" by constructing a right parallelepiped of height φ' on a rectangular base of dimensions φx1. Four of its six surfaces are golden rectangles; the other two are rectangles of dimension φxφ'. Many of the interesting features of the golden rectangle (e.g., embedded self-similarity) can be extended to three dimensions with the golden solid. One of its most surprising features is that the ratio of the surface area of a sphere circumscribing a golden solid to the surface area of the golden solid itself, is φ!

The regular pentagon has traditionally been put forward as a source of many examples of φ as well. For instance, any diagonal of the pentagon, where the side is taken as the unit, has a length of φ. Also, the diagonal of any regular pentagon divides any other diagonal of the pentagon into a φ ratio (Figure 5a). If a regular pentagram is constructed out of such a pentagon by extending its sides until they intersect each other (Figure 5b), the result contains many instances of φ as well. Taking the length of a side of the original pentagon as the unit, the lengths of the sides of the points of the pentagram beyond the boundaries of the original pentagon are of length φ. The distance from the tip one point to the tip of an adjacent one is φ². The distance through the pentagram from one point tip to another two points away is φ³.

Now notice that each point of the pentagram forms an isosceles triangle that is 72° at the base and 36° at the apex. The same triangle is formed by any two interior diagonals of the pentagon that meet at the same angle and the opposite side. This triangle is a rich source of examples of φ in its own right; so much so that it is known as the "golden triangle" (Figure 6a). To begin with, the ratio of the lengths of each of its legs to that of its base is φ. It is important to note that the ratio of its height to its base is not φ, an erroneous assumption frequently made by contemporary psychological researchers. A line equal in length to the base, drawn from the apex of the base and one leg across the triangle to meet with the opposite leg divides the second leg in a golden section (Figure 6b). Further, the triangle formed below the new line is itself a golden triangle. By repeating this process with progressively smaller figures, golden triangles can be embedded in each other to form an infinitely deep fractal, in a manner similar to that used with the golden rectangle. Finally, connecting all the corners of the a set of embedded golden triangles with a spiral curve results in a logarithmic spiral, just as it did with the whirling squares. Another interesting detail is that φ is hidden even in the component angles of the golden triangle: the cosine of 36° is φ/2, and the cosine of 72° is φ'/2.

Recall that φ' is the answer to the question, "what division of a line gives a ratio of the shorter segment to the longer equal to the ratio of the longer segment to the whole?" One can ask a similar question for angles: "what angular division of a circle gives a ratio of the smaller angle to the larger angle identical to the ratio of the larger angle to the whole circle?" In more formal terms:

The answer to this question, perhaps hardly surprisingly by now, involves φ: b = 360°/φ (≈222.5°) and a = b/φ (≈137.5°). Correspondingly, a has come to be known as the "golden angle", or "ideal angle."

There are many other geometrical instances of φ. The standard reference used by most psychological researchers is Huntley (1970). More recently a very detailed and sophisticated account by Herz-Fischler (1987/1998) has been published. Another excellent account that includes links to contemporary work on fractals and tiling is Kappraff (1991). The question remains, however, what interest might this all be to the non-mathematician? One answer is that there appear to be many examples of φ in the natural world as well.

Pentagonal symmetry is quite common in the natural world, particularly among the "lower" phyla. Because φ is so intimately connected with the pentagon, as discussed above, this has often been taken as a starting point for investigations of the "natural reality" of φ. Flowers of many kinds exhibit pentagonal symmetry. Ghyka (1946/1977, p. 18, n.) cites "all fruit-blossoms, water-lilies, brier-roses and all the genus rosa, honeysuckle, carnations, geraniums, primroses, marsh-mallows, campanula, passion-flowers, et cetera." Berlyne (1971, p. 224) says that pentagonal symmetry is characteristic of the flowers of the dicotyledonous angiosperms. A wide variety of sea creatures also exhibit pentagonal symmetry. Of particular note, says Ghyka (1946/1977, p. 18) are the starfish, the jellyfish, and the sea urchins. Hargittai (1992) has edited an entire book on pentagonal symmetry in nature.

Another connection between φ and the natural world is in the formation of shells. As is often pointed out, the shell of the Nautilus is a logarithmic spiral, but the growth patterns of many other shells -- e.g., of the abalone and the triton -- also show the same sort of geometric growth pattern characteristic of the logarithmic spiral (see Ghyka, 1946/1977, pp. 93-97). In addition, the logarithmic spiral is characteristic of the patterns of growth found on pinecones, pineapples, and sunflowers.

A third link between the natural world and φ is the frequency with which Fibonacci series are seen in nature. Recall that the ratios of adjacent numbers in all Fibonacci series asymptote to φ. Fibonacci (Leonardo of Pisa, ca. 1175-1250) himself showed that the growth of a rabbit population can be modeled by a Fibonacci series. The same is true of the growth of a beehive population, even though the reproductive principles for bees are quite different (viz., drones are born of unfertilized eggs). The relation between Fibonacci numbers and phyllotaxis (the study of the arrangements of leaves on plants) is also widely cited, but statements of this claim are traditionally chock through with myths, half-truths, and misconceptions. Ghyka (1946/1977) claims that, "Church ... discovered that [the golden angle] corresponds to the best distribution of leaves" (p. 16), where "best" is explicated as "a constant angle between leaves or branches on a stem producing the maximum exposition to vertical light" (p. 14). He gives no exact reference, however. Ghyka then goes on to say that "the mathematical confirmation [of Church's discovery] was given by Wiesner in 1875" (p. 16), but, again, gives no exact reference. Huntley (1970, pp. 161-163) says that it has been shown that leaf divergence follows a Fibonaccian pattern characteristic of each plant. He relies, however, on a popular account of mathematical games by H. E. Licks. Huntley also describes the increase in axils on the stem of a developing plant as a Fibonaccian process, but give no reference. In a similar vein, Berlyne (1971, p. 224) claims that "approximations to φ are found in the number of grains encircling a pine cone in two perpendicular directions, and D'Arcy Thompson (1917) has shown mathematically why this must be the case."

More recent work by the botanist G. J. Mitchison (1977), however, has called the accuracy of these claims into some question. According to him, there are two main sorts of phyllotaxic patterns: (1) decussate -- in which pairs of leaves sprout on opposite sides of the stem, and successive pairs grow at right angles to each other -- and (2) spiral. Each petal or leaf of a plant that exhibits a spiral phyllotaxic pattern makes contact with exactly two other leaves of the next inner pass of the spiral. Consequently, depending on which contact leaf one follows, one can trace sets of spirals, called "parastichies", in both clockwise and counterclockwise patterns. What Church (1904) discovered is that the number of parastichies, clockwise and counterclockwise, in a given plant are numbers in a Fibonacci series (e.g., five and eight in a pine cone, 89 and 144 in a sunflower, respectively). Since they follow Fibonaccian patterns, their ratios approximate φ.

Mitchison (1977, p. 271) also argues that Thompson's "proof" that plants are mathematically constrained to follow such patterns is invalid, "for it is perfectly possible to construct a lattice with any chosen contact numbers m and n, or any chosen divergences angle from 0° to 180°. The answer [to why such a pattern appears] is to be found in the way the plant grows." Briefly, the initial leaves are often 180° apart. But as new leaves develop, they are positioned so as to be in contact with two older leaves. Because the stem thickens as it matures, Mitchison explains, "the pitch of the genetic spiral [i.e., the pattern of all leaves in the order of their genesis, not the "parastichy" spiral of contacting leaves, described above] must decrease" (p. 271). The result of this process is that angular divergence of new leaves gradually approximates the golden angle. This gives rise to an approximate logarithmic spiral of touching leaves.

Finally, there are claimed to be a wide array examples of the golden section itself in mammalian anatomy. These claims are more controversial than those previously described because they involve notions of the "ideal" human, and because the actual research is quite old, its details being difficult to track down. Ghyka (1946/1977, pp. 98-708) argues, based on research much of which he does not cite explicitly, that φ is characteristic of a great number of features of the "ideal" human body. For instance, (1) the ratio of overall height to the height of the navel, (2) the ratio of the height below the navel to that above it, and (3) the ratio of the height above the navel to that between the navel and the chin, are all said to be equal to φ. Even the three bones of the finger are said to grow in a ratio of 1, 1/φ, 1/φ² (Ghyka 1946/1977, p. 16). Among the scholars Ghyka mentions, Hambidge is said to have analysed the measurement of "hundreds" of human skeletons and, "although his individual measurements vary ... each skeleton reveals what is a perfect 'symphonic' design" (Ghyka, 1946/1977, p. 97). Many ratios of features of the "ideal" human face are also claimed to be "golden." For instance, the ratio of the height of the whole head to that of the head above the nose, is said to be equal to φ. The racist overtones historically associated with research into what constitutes the "ideal" human -- Zeising's views, for instance, seem to have been explicitly so -- make this sort of work unpalatable to many contemporary scholars. At least one scholar, however, still holds that the perceived beauty of the golden section is derived from the natural ratios of the human female face (Fensome, 1981).

Ghyka claims, however, that "other examples can be found all through the animal world (including insects), and the resulting diagrams of proportions, however diversely arranged, can be deciphered by the same key" (p. 108). He presents, as an example, a "harmonic analysis" of thoroughbred horse in which ratios such as the length of the leg to the vertical thickness of the body are φ. Such claims should not be viewed without a healthy degree of skepticism in the absence of full and replicated scientific reports, but neither is there reason to dismiss them out of hand.

Biology is not the only natural science in which Fibonacci series are to be found. In certain aspects of physics, they arise as well. Imagine that two sheets of glass are put back to back and a beam light is shown on them. There are four reflecting surfaces (the front and back surface of each sheet. The possible patterns of reflection are endless, but for any given number of reflections, Huntley (1970, pp. 154-155) shows that the number of possible patterns of reflection is a Fibonacci number (e.g., 3 patterns of two reflections, 5 patterns of three reflection, 8 patterns of four, etc.). Huntley (1970, pp. 156-157) also shows that the history of energy levels of the electron of the hydrogen atom can be modeled by a Fibonacci series as well.

The discovery of φ seems to have been quite ancient. This section traces its history, from the time of Egyptian pre-eminence to the 19th century.

It is typical to credit the Egyptians with all manner of arcane mathematical knowledge (see, e.g., Ghyka (1946/1977, pp. 60-68). Discovery of the "Pythagorean" theorem, π, and φ are just a few of the breakthroughs they are said to have made. It is often implied, if not stated outright, that Euclid's geometry was really lifted almost verbatim from the texts of the architects of the pyramids. Myth and half-truth abound in accounts of the alleged transmission of such mathematical knowledge as the ancient Egyptians had to the early Greek philosophers. It is often claimed that Thales or Pythagoras or both travelled to Egypt and acquired their geometrical secrets (see, e.g., Heath, 1920; Turnbull, 1929/1961; Kappraff, 1991). Diamandopoulos (1967, p. 97) says that, on the evidence, such claims "must be judged as unhistorical," and Neugebauer (1962, p. 142) ridicules them as "amusing fairy tales." Gorman (1979, p. 39), on the other hand, doubts neither story, and baldly states that "Pythagoras...was twenty-two when he made the decision to go to Egypt." This is not the appropriate venue for a detailed investigation of these claims. The only caveat I will convey to the interested reader is that most of the claims, both true and false, are passed on uncritically from one writer to another. Fairly few actually refer to the original documents whence the claims originate. Kappraff (1991) refers to Turnbull (1929/1961) and Gorman (1979). Turnbull's material, in turn, seems to be almost entirely drawn from Heath (1920). Heath (1920) and Gorman (1979) both cite specific ancient documents, and therefore are reasonable starting points for the study of Thales' and Pythagoras' contributions (though some of Heath's material is now out of date). On the state of Egyptian and Babylonian mathematical knowledge, the most reliable sources are Neugebauer (1962) and Gillings (1972/1982). Ghyka's (1946/1977) work is poorly referenced, and where it is adequately documented, sometimes misconstrues the significance of some work. Huntley (1970) is less tendentious, but not much better with references.

To summarize the situation as fairly as possible, it seems that the mathematical knowledge of the Ancient Egyptians, though not inconsiderable, was largely practical rather than theoretical. It is fair to say that (1) they had an estimate of φ accurate within 0.5%, (2) some religious buildings might have been built with φ in mind, but these may also be artifacts of their building techniques, and (3) they seem to have employed a building technique called "rope stretching", that relied on the truth of the Pythagorean theorem. None of this seem to have been achieved with the assistance of abstract mathematics, however. The irrationality of numbers like φ, π, and √2 never seems to have dawned on them, nor is it likely that it would have had much importance for them if it did. Proofs were never given, although some justification was occasionally offered. Largely, just the bare facts of the matter -- sophisticated "rules of thumb" for the most part -- were passed on from scribe to scribe and architect to architect. On this basis, one might argue that Egyptian mathematicians were like Levi-Strauss' bricoleurs (though on a much grander scale); concrete scientists who develop the applied knowledge they require without extending it into a full theoretical discipline.

As for the transmission of mathematical knowledge to Greece, either Thales or Pythagoras may have gone to Egypt, and Pythagoras may have gone to Babylon, but there seems to be little in the way of direct evidence to verify these claims. Greek and, later, Roman historians made such claims routinely, but the "secret wisdom" of Egypt, a kingdom ancient even to them, seems to have been an unquestioned assumption of the Greeks world view. Neither the Greeks nor the Egyptians seem motivated to doubt that a discipline as arcane as geometry must have come down from time immemorial, and to their minds that implied an origin in the Nile Valley.

Regardless of the mode of transmission, there is no doubt that the Classical Greeks possessed knowledge of, and a fascination with, the golden section. Proclus reported that the Greek geometers of the Platonic schools called it simply hê tomê, "the section" (cited in Ghyka 1946/1977, p. 4). Euclid discussed it extensively, calling it the "division into mean and extreme ratio" (cited in Berlyne, 1971, p. 222). Hambidge (1920) has shown that the shapes of Classical Greek vases are based on a set of related "dynamic" forms that include the golden rectangle. Most famous of all, the face of the Parthenon, designed by Phidias, originally possessed almost exactly "golden" proportions. Further, the aesthetic valuation of the golden section, and like ratios, was transmitted to the Romans as well (See Ghyka, 1946/1977, p. 5; Kappraff, 1991, chap. 1; Watts & Watts, 1986). Most notable of these was the great architect Vitruvius, whose works formed the basis of Medieval architecture as well as Roman.

It is customary to cite Alberti's De re aedificatoria (1485) when discussing the influence of the golden section on Medieval and Renaissance architects and artists. But Alberti neither invented anew nor "rediscovered" the architectural principles regarding the golden section described there. It would be more correct to characterize his work as a codification or formalization, albeit an elegant one, of principles that had been handed down from mason to mason for centuries. The evidence is that Mathes Roriczer's Geometria Deutsch and Lorenz Lechler's Instructions -- northern manuals on architecture of the same period--explained similar technical skills (Coldstream, 1991, p. 33). Further, gothic cathedrals, though they underwent radical stylistic changes, were based on the same architectural principles in the 16th century as they had been when they first rose in the 12th.

For reasons that are easy to discern, the golden rectangle and the √2 rectangle, in particular, were among the favorite shapes of the master builders of this era . It is often insinuated that this preference was due to some sort of religiously-motivated Medieval numerological mysticism. Although it is true that Medieval treatises on beauty made a great deal of the terms claritas and consonantia (clarity or simplicity, and harmony or consonance, respectively), an even cursory look at their construction techniques reveals a more realistic, less pejorative, explanation.

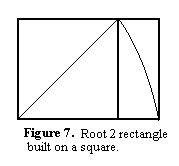
Recall that the golden rectangle can be constructed by swinging an arc, centered at the midpoint of the bottom of a square, from the upper corner to a point collinear with the bottom line (Figure 2b). One can similarly construct a √2 rectangle by swinging an arc, centered on a lower corner, from the diagonally opposite upper corner to a point collinear with the bottom side of the square (see Fig 7.).Now, imagine that one wants to build an arch over a building that, in cross-section, is a 2:1 rectangle. If one swings an arc, centered on the bottom center of this rectangle, from one upper corner to the other, the resulting arc is √2 units high. The arc is quite low, however. If one, alternatively, centers the arc on the midpoint of the rectangle, the arc soars much higher. In fact, it is precisely φ units above the ground. The same technique can be used to inscribe an apse attached to a square or rectangular building as well.

It is true, as is often pointed out, that the √2 arch, and the whole building below it, fits exactly in a perfect semicircle and that the φ arch and the building below it fits exactly into a perfect circle, and that this sort of architectural "harmony" appealed strongly to both the Medieval and the Renaissance mind. However, the great advantage of such design techniques was that they could be executed by people who had minimal knowledge of engineering or geometry. A piton could be driven into the desired center point, and a rope used the inscribe the arc to be built upon. No measurement or calculation was required. Thus, techniques much like this could be executed by underling builders, freeing the master mason for other more pressing duties.

I would, thus, speculate that the fact that such simple and serviceable construction techniques resulted in such basic mathematical relations was, at least in part, the historical cause of Classical artists' and architects' fascination with such relations, rather than a prior fascination with such relations being the cause of their incorporation of them into their works. By the time the Middle Ages rolled around, the two ideas had become inextricably intertwined. Consider whether the fascination with √2 and φ would have been so great had the simple geometrical techniques described above had resulted in, say, arches of height e (2.718...). It is doubtful.

By the 16th century, interest in the golden section was widespread enough that Luca Pacioli di Borgo published a treatise about it entitled De Divina Proportione (1509), illustrated by none other than Leonardo da Vinci. Consequently, "The Divine Proportion" became the name by which the ratio was best known. At the end of the same century, a then young mathematics instructor, Johannes Kepler (1597), wrote in his Mysterium Cosmographicum:

The neo-Platonism of the age supported, and was supported by, the interest in the myriad and fascinating properties and uses of the golden section. With the rise of empiricism, however, in the 17th century, interest in such matters came to be actively discouraged. As is true in most revolutions, whether political or intellectual, babies are thrown out with bathwater and, "babies" such as the golden section likely came to be frowned upon as idle numerology, "Scholascticism" (used pejoratively), and formalism. It is worth noting that even logic itself came to be disdained during this period. According the Kappraff (1991, p. 16), it was just this defeat of mathematical aesthetic theories of that led to "a state of confusion" in architecture and, ultimately, to the rise of aesthetic subjectivism -- usually read: "hedonism" -- that so many today believe to be the only possible basis of aesthetic theory.

It would not be until the 19th century that significant interest in the golden section (and logic) was revived, and not until the 20th century that LeCorbusier would again make φ the centerpiece of architectural theory in Modulor. The most significant figure in the 19th century revival of interest in φ advocate was, as mentioned above, Adolf Zeising. It was his works, at least in part, that stimulated G. T. Fechner to select the golden rectangle as one the first stimulus objects to be used in a scientific psychological study.