Overview
This is the 4th meeting in an international conference series on Dynamics of Differential Equations - in Memory of Jack K. Hale. The conference series was established through the joint effort of research groups in dynamics of differential equations from across the Americas, Asia, and Europe, with scientific focus on the advancement and application of dynamical systems as generated by differential equations and their discrete analogs. The series has had successful meetings in Atlanta, USA (2013), Luminy, France (2016), and Hiroshima, Japan (2018).
The 4th meeting will consist of:
● Two Hale Memorial Lectures delivered by Professor John Mallet-Paret;
● One Plenary Session with 10 plenary lectures;
● One Special Session on General Dynamics;
● One Workshop on Dynamics of Infectious Diseases; and
● One Workshop on Dynamics and Evolutionary Equations.
This latter workshop is in honor of Professor Genevieve Raugel, who was a leading expert in the area and also a co-founder of this conference series.
Besides carrying on the general theme of the series, this 4th meeting will give emphasis to the development of emerging areas of dynamics, including stochastic dynamics and the connection of dynamics with data science applications.
Full Schedule
G: Special Session on General Dynamics,
A: Workshop on Dynamics of Infectious Diseases;
R: Workshop on Dynamics & Evolution Equations - in honor of Geneviève Raugel;
Xij: ith (1,2,3,4) day, jth (1,2,3,4) talk in the parallel sessions G, A, and R.
August 14, Monday
| Special Session on General Dynamics | Workshop on Dynamics of Infectious Diseases | Workshop on Dynamics & Evolutionary Equations - in honor of Geneviève Raugel | |
| Chair | Jianhong Wu | Jacques Belair | Yingfei Yi |
| 2:00- 2:40 | G11 Odo Diekmann | A11 Zhilan Feng | R11 Luca Dieci |
| 2:40-3:20 | G12 Roman Srzednicki | A12 Shigui Ruan | R12 Hermen Jan Hupkes |
| 3:20-3:40 | Coffee Break | ||
| Chair | Huaiping Zhu | Michael Li | Arnd Scheel |
| 3:40-4:20 | G13 Yihong Du | A13 Monica Cojocaru | R13 Hans-Otto Walther |
| 4:20-5:00 | G14 Cinzia Elia | A14 Yu Jin | R14 Tomas Gedeon |
August 15, Tuesday
| Chair | Tibor Krisztin | Zhilan Feng | Eugene Wayne |
| 2:00- 2:40 | G21 Junya Nishiguchi | A21 Gyllenberg Mats | R21 Arnd Scheel |
| 2:40-3:20 | G22 Lu Xu | A22 Zhigui Lin | R22 Dmitry Pelinovsky |
| 3:20-3:40 | Coffee Break | ||
| Chair | Christiane Rousseau | Monica Cojocaru | Weishi Liu |
| 3:40-4:20 | G23 Xiaoying Han | A23 Jane Marie Heffernan | R23 Erik Van Vleck |
| 4:20-5:00 | G24 Elena Braverman | A24 Yuan Yuan | R24 Xingfu Zou |
August 16, Wednesday
| Chair | Gail Wolkowicz | Gyllenberg Mats | Jianhong Wu |
| 2:00- 2:40 | G31 Sue Ann Campbell | A31 Xiaoqiang Zhao | R31 Carmen Nunez |
| 2:40-3:20 | G32 Zhenxin Liu | A32 Kunquan Lan | R32 Bernhard Lani-Wayda |
| 3:20-3:40 | Coffee Break | ||
| Chair | Odo Diekmann | Luca Dieci | Peter Polacik |
| 3:40-4:20 | G33 Yao Li | A33 Xinzhi Liu | R33 Weishi Liu |
| 4:20-5:00 | G34 Susmita Sadhu | A34 Muhammad Arif | R34 Marcio Gameiro |
August 17, Thursday
| Chair | Wen Huang | Shigui Ruan | Hans-Otto Walther |
| 2:00- 2:40 | G41 Zhongwei Shen | A41 Michael Li | R41 Phillipo Lappicy |
| 2:40-3:20 | G42 Weiwei Qi | A42 Chunhua Shan | R42 Montie Avery |
| 3:20-3:40 | Coffee Break | ||
| Chair | Hans-Otto Walther | Michael Li | Raphael Krikorian |
| 3:40-4:20 | G43 Shangbing Ai | A43 Yukihiko Nakata | R43 Roberta Fabbri |
| 4:20-5:00 | G44 Shirou Wang | A44 Pei Yuan | R44 Yingfei YI |
Program
Monday August 14
| 8:45- 9:00 | Opening | |
| 9:00-9:50 | Chair Luca Dieci | Hale lecture I by John Mallet-Paret Analyticity in Delay-Differential Equations |
| 9:50-10:10 | Coffee Break | |
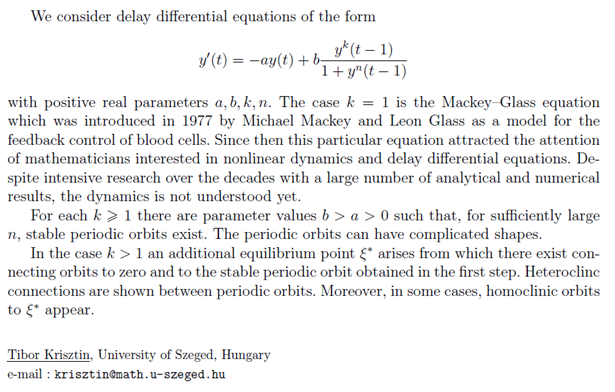
| 10:10-11:00 | Chair Hans-Otto Walther | Plenary lecture, Tibor Krisztin Periodic and connecting orbits for Mackey–Glass type equations |
| 11:00-11:50 | Plenary lecture, Jianhong Wu Assorted problems of delay differential equations modeling impact of individual behavioral adaptation on disease transmission in the population | |
Tuesday, August 15
| 9:00-9:50 | Chair Xiaoqiang Zhao | Hale lecture II by John Mallet-Paret Genericity in Delay-Differential Equations |
| 9:50-10:10 | Coffee Break | |
| 10:10-11:00 | Chair Jacques Belair | Plenary lecture, Michael Mackey Mathematical models of gene regulation: Biology drives new mathematics |
| 11:00-11:50 | Plenary lecture, Wenxian Shen Dynamical aspects of chemotaxis models with logistic source on the whole space |
Wednesday, August 16
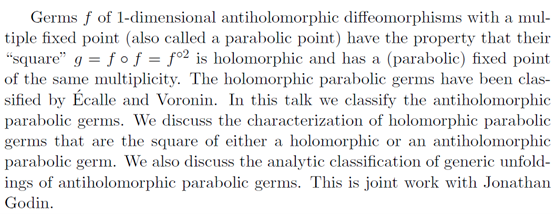
| 9:00-9:50 | Chair Sue Ann Campbell | Plenary lecture Christiane Rousseau Parabolic points in antiholomorphic and holomorphic dynamics |
| 9:50-10:10 | Coffee Break | |
| 10:10-11:00 | Chair Yingfei Yi | Plenary lecture, Raphael Krikorian Divergence and convergence of Birkhoff Normal Forms |
| 11:00-11:50 | Plenary lecture, Wen Huang Full horseshoe for the Galerkin truncations of 2D Navier-Stokes equations with degenerate stochastic forcing |
Thursday, August 17
| 9:00-9:50 | Chair Huaiping Zhu | Plenary lecture, Eugene Wayne Breathers and the Implicit Function Theorem |
| 9:50-10:10 | Coffee Break | |
| 10:10-11:00 | Chair Michael Li | Plenary lecture, Gail S. K. Wolkowicz Modeling Delay in Population Growth Differential and Difference Equation Models |
| 11:00-11:50 | Plenary lecture, Peter Polacik On the large-time behavior of bounded solutions of semilinear parabolic equations on R^N |
August 14, Monday
| Chair | Jianhong Wu | |
| 2:00- 2:40 | G11 Odo Diekmann On the formulation and analysis of size-structured consumer resource models | |
| 2:40-3:20 | G12 Roman Srzednicki On determining the existence of periodic orbits | |
| 3:20-3:40 | Coffee Break | |
| Chair | Huaiping Zhu | |
| 3:40-4:20 | G13 Yihong Du Exact rate of acceleration in propagation determined by KPP equations with nonlocal diffusion and free boundaries | |
| 4:20-5:00 | G14 Cinzia Elia Synchronization of piecewise-smooth networks | |
August 15, Tuesday
| Chair | Tibor Krisztin | |
| 2:00- 2:40 | G21 Junya Nishiguchi “Mild solutions” and variation of constants formula for delay differential equations | |
| 2:40-3:20 | G22 Lu Xu KAM Theorems in application for multi-scale systems | |
| 3:20-3:40 | Coffee Break | |
| Chair | Christiane Rousseau | |
| 3:40-4:20 | G23 Xiaoying Han Dynamics of lattice models arising from neural networks | |
| 4:20-5:00 | G24 Elena Braverman On stability and asymptotics of systems with distributed unbounded delays | |
August 16, Wednesday
| Chair | Gail Wolkowicz | |
| 2:00- 2:40 | G31 Sue Ann Campbell Dynamics of a diffusive nutrient phytoplankton-zooplankton model with spatio-temporal delay | |
| 2:40-3:20 | G32 Zhenxin Liu The averaging principle for SDEs with monotone coefficients | |
| 3:20-3:40 | Coffee Break | |
| Chair | Odo Diekmann | |
| 3:40-4:20 | G33 Yao Li Data-driven Computation of Stochastic Dynamics | |
| 4:20-5:00 | G34 Susmita Sadhu Analysis of long transient dynamics and detecting early warning signals of regime shifts in a class of predator-prey models featuring two timescales | |
August 17, Thursday
| Chair | Wen Huang | |
| 2:00-2:40 | G41 Zhongwei Shen Stochastic stability of invariant measures | |
| 2:40-3:20 | G42 Weiwei Qi Long transient dynamics in stochastic systems with applications | |
| 3:20-3:40 | Coffee Break | |
| Chair | Hans-Otto Walther | |
| 3:40-4:20 | G43 Shangbing Ai Relaxation oscillations in predator-prey systems | |
| 4:20-5:00 | G44 Shirou Wang Can one hear the shape of high-dimensional landscape? | |
August 14, Monday
| Chair | Jacques Belair | |
| 2:00- 2:40 | A11 Zhilan Feng Analysis of metapopulation models of the transmission of SARS-CoV-2 in the United States | |
| 2:40-3:20 | A12 Shigui Ruan Age-structured population dynamics with nonlocal diffusion | |
| 3:20-3:40 | Coffee Break | |
| Chair | Michael Li | |
| 3:40-4:20 | A13 Monica Cojocaru Forecasting individual and group decisions using game theory, projected systems and Kalman filtering | |
| 4:20-5:00 | A14 Yu Jin Spatial dynamics of a pest population with stage-structure and control | |
August 15, Tuesday
| Chair | Zhilan Feng | |
| 2:00- 2:40 | A21 Gyllenberg Mats | |
| 2:40-3:20 | A22 Zhigui Lin West Nile virus diffusive models with warming and seasonal succession | |
| 3:20-3:40 | Coffee Break | |
| Chair | Monica Cojocaru | |
| 3:40-4:20 | A23 Jane Marie Heffernan Modelling Immunity | |
| 4:20-5:00 | A24 Yuan Yuan Bifurcation in a diffusive predator-prey model with discontinuous harvesting | |
August 16, Wednesday
| Chair | Gyllenberg Mats | |
| 2:00- 2:40 | A31 Xiaoqiang Zhao The Linear Stability and Basic Reproduction Numbers for Autonomous FDEs | |
| 2:40-3:20 | A32 Kunquan Lan Existence &uniqueness of nonlinear Cauchy problems for first order fractional DEs | |
| 3:20-3:40 | Coffee Break | |
| Chair | Luca Dieci | |
| 3:40-4:20 | A33 Xinzhi Liu Hybrid Formation Control of Multi-Agent Systems with Time Delays | |
| 4:20-5:00 | A34 Muhammad Arif The dynamics of COVID-19 epidemic in Pakistan using SEIR model & prediction | |
August 17, Thursday
| Chair | Shigui Ruan | |
| 2:00- 2:40 | A41 Michael Li Re-thinking the Mathematical Theory of Epidemics | |
| 2:40-3:20 | A42 Chunhua Shan Complex dynamics of epidemic models on adaptive networks | |
| 3:20-3:40 | Coffee Break | |
| Chair | Michael Li | |
| 3:40-4:20 | A43 Yukihiko Nakata An epidemic model for reinfection dynamics with heterogeneous susceptibility | |
| 4:20-5:00 | A44 Pei Yuan Predictive modelling and forecasting of the mosquito abundance and risk of West Nile virus | |
August 14, Monday
| Chair | Yingfei Yi | |
| 2:00- 2:40 | R11 Luca Dieci Semi-discrete optimal transport problems | |
| 2:40-3:20 | R12 Hermen Jan Hupkes Stochastic speed and shape corrections for travelling patterns in random media | |
| 3:20-3:40 | Coffee Break | |
| Chair | Arnd Scheel | |
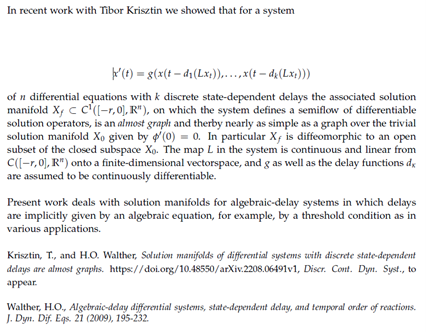
| 3:40-4:20 | R13 Hans-Otto Walther On solution manifolds | |
| 4:20-5:00 | R14 Tomas Gedeon Combinatorial structure of continuous dynamics in gene regulatory networks | |
August 15, Tuesday
| Chair | Eugene Wayne | |
| 2:00- 2:40 | R21 Arnd Scheel Propagation into unstable states: linear predictions and nonlinear results | |
| 2:40-3:20 | R22 Dmitry Pelinovsky Analysis and modeling of the modular Burgers equation | |
| 3:20-3:40 | Coffee Break | |
| Chair | Weishi Liu | |
| 3:40-4:20 | R23 Erik Van Vleck Projection Techniques and Reduced Order Modeling for Data Assimilation | |
| 4:20-5:00 | R24 Xingfu Zou Modelling the impact of human precaution on disease dynamics and its evolution | |
August 16, Wednesday
| Chair | Jianhong Wu | |
| 2:00- 2:40 | R31 Carmen Nunez Critical transitions in nonautonomous ordinary equations with concave derivative | |
| 2:40-3:20 | R32 Bernhard Lani-Wayda Linearized instability for differential equations with dependence on the past derivative | |
| 3:20-3:40 | Coffee Break | |
| Chair | Peter Polacik | |
| 3:40-4:20 | R33 Weishi Liu Permanent charge effects on ionic flows | |
| 4:20-5:00 | R34 Marcio Gameiro Computing the Global Dynamics of Parameterized Systems of ODEs | |
August 17, Thursday
| Chair | Hans-Otto Walther | |
| 2:00- 2:40 | R41 Phillipo Lappicy Disturbing the Big Bang | |
| 2:40-3:20 | R42 Montie Avery Universal dynamics of invasion fronts | |
| 3:20-3:40 | Coffee Break | |
| Chair | Raphael Krikorian | |
| 3:40-4:20 | R43 Roberta Fabbri A global bifurcation diagram for a one-parameter family of nonautonomous scalar ODE's driven by a minimal flow | |
| 4:20-5:00 | R44 Yingfei Yi Response solutions in degenerate, quasi-periodically forced nonlinear oscillators | |
Titles and Abstracts
Hale memorial lectures

John Mallet-Paret, Brown University, USA
LECTURE 1: Analyticity in Delay-Differential Equations
We study classes of delay-differential equations for which the nonlinearities in the equations are analytic functions. One might expect (as with ordinary differential equations) that many solutions, particularly those on the attractor, are analytic functions of time. This is often, but not always, true. We discuss various theorems and examples which illustrate such phenomena.
LECTURE 2: Genericity in Delay-Differential Equations
As noted in the first lecture, solutions of analytic delay-differential equations need not be analytic. Yet numerical simulations of such systems (often with parameters) typically present an analytic picture, with smooth branches of solutions and simple bifurcations. In this spirit we study generic properties of smooth (but not necessarily analytic) delay-differential equations.
Plenary lectures
Title: Full horseshoe for the Galerkin truncations of 2D Navier-Stokes equations with degenerate stochastic forcing
Abstract: In this talk, we will introduce the existence of full horseshoe for the Galerkin truncations of 2D Navier-Stokes equations with degenerate stochastic forcing (Hypoelliptic condition). We will also review weak horseshoe and semi-horseshoe. This is based on joint work with Jianhua Zhang.
Title: Divergence and convergence of Birkhoff Normal Forms.
Abstract: Each real analytic symplectic diffeomorphism admitting a non-resonant fixed point can be formally conjugated to a formal integrable symplectic diffeomorphism, its Birkhoff Normal Form (BNF). I shall discuss two questions. The first one is due to H. Eliasson: are there examples of divergent BNF? The second is the following: is it possible to perturb in the real analytic topology a given real analytic symplectic diffeomorphism so that its BNF converges?
Title: Periodic and connecting orbits for Mackey–Glass type equations
Title: Mathematical models of gene regulation: Biology drives new mathematics
Abstract: Simple bacterial gene regulatory motifs can be viewed from the perspective of dynamical systems theory, and mathematical models of these have existed almost since the statement of the operon concept. In this talk I will review the three basic types of these regulatory mechanisms and the underlying dynamical systems concepts that apply in each case. In the latter part of the talk, I will discuss the exciting mathematical challenges that arise when trying to make honest mathematical models of the underlying biology. These include transcriptional and translational delays, and the fact that these delays maybe state dependent, as well as the interesting and often unsolved problems of characterizing the noise inherent in bacterial dynamics. Surprisingly, the inclusion of state dependent delays can have rather profound effects on the possible dynamical behaviour, and it is unclear if this is reflected in biological behaviour.
Title: On the large-time behavior of bounded solutions of semilinear parabolic equations on R^N
Abstract: We will mainly consider reaction-diffusion equations on the real-line (N=1), adding some results concerning radial solutions in higher space dimensions. Our main focus is the quasiconvergence of bounded solutions, that is, locally uniform convergence of bounded solutions to a set of steady states. While not all bounded solutions have this property in general, we identify a large class of initial data which do give rise to quasiconvergent solutions.
Title: Parabolic points in antiholomorphic and holomorphic dynamics
Title: Breathers and the Implicit Function Theorem
Abstract: Breather solutions are spatially localized, temporally periodic solutions of partial differential equations and lattice systems. They are extremely rare for partial differential equations with constant coefficients but are known to exist in some PDEs with inhomogeneous coefficients. In this talk I’ll describe a new approach to the construction of breathers for special classes of PDEs with spatially periodic coefficients, based on the implicit function theorem.
Title: Dynamical aspects of chemotaxis models with logistic source on the whole space
Abstract: Chemotaxis models are used to describe the movements of mobile species or living organisms in response to certain chemicals in their environments. The current talk is concerned with various dynamical aspects of chemotaxis models with logistic source on the whole space. I will first discuss the global existence of positive classical solutions of such chemotaxis models, which is fundamental for the study of various dynamical aspects. Next, I will discuss the asymptotic behavior of globally defined positive solutions with strictly positive initial functions. I will then consider the spatial spreading dynamics of positive solutions with compactly supported or front-like initial functions.
Title: Modeling Delay in Population Growth Differential and Difference Equation Models
Abstract: Depending upon the underlying assumptions and reason for including delay in a model of population growth, different strategies for deriving the models will be discussed. Discrete delay differential equations, integro-differential equations and difference equations with both discrete and distrbuted delay will be considered. In all cases the delay is included in the growth of the population consistent with the decline of the population predicted by the model so that those contributing to the growth survive the delay period. In each case there is an important critical delay threshold that depends on the length of the delay and other parameters in the model. If the length of the time delay exceeds this threshold, the models predict that the population will go extinct for any non-negative initial conditions. Below this threshold, there is at least one positive equilibrium. The number of positive equilibria, their stability, and how their magnitude depends on the length of the delay will be discussed.
Title: Assorted problems of delay differential equations modeling impact of individual behavioral adaptation on disease transmission in the population
Abstract: I will introduce a few classes of delay differential equations/renewal equations arising from viral infection spread vector-borne disease spread in the respective populations. These equations have some particular components for individual behaviors, leading to renewal and delay equations governing the behavioural adaptation dynamics. I will talk about some preliminary results on the qualitative properties (multiple waves and multi-stability) of the disease spread and behavioural adaptation coupled syst
Special Session on General Dynamics
Title: Relaxation oscillations in predator-prey systems
Abstract: We will present some recent existence results on relaxation oscillations in predator-prey systems. We will outline their proofs that depend on entry-exit functions and positively invariant annular domains near some singular closed orbits. The talk is based on joint works with Susmita Sadhu and Yingfei Yi.
Title: On stability and asymptotics of equations and systems with distributed unbounded delays
Abstract: Many differential equations of mathematical biology assume delayed production process and the instantaneous mortality. Introduction of delay can destabilize the unique positive equilibrium and even lead to chaos. However, for some types of equations and systems, lags in the reproduction term do not change stability properties. Consideration of variable, unbounded and distributed delays emphasizes robustness of this `absolute stability' property. Influence of an infinite, not just unbounded, delay is also outlined.
Title: Dynamics of a Diffusive Nutrient Phytoplankton-Zooplankton Model with Spatio-Temporal delay.
Abstract: We study a diffusive nutrient-phytoplankton-zooplankton (NPZ) model with spatio-temporal delay. The closed nature of the system allows the formulation of a conservation law of biomass that governs the ecosystem. We formulate stability conditions for the equilibria for a general distribution of delays and analyze the Hopf bifurcations for a specific delay kernel. We show that diffusion predominantly has a stabilizing effect; however, if sufficient nutrient is present, complex spatio-temporal dynamics, both transient and long lasting may occur.
Title: On the formulation and analysis of size-structured consumer resource models
Abstract: To describe the dynamics of a size-structured population and its unstructured resource, we formulate bookkeeping equations in two different ways. The first, called the PDE formulation is by far the most natural one. It employs a first order partial differential equation, with a non-local boundary condition, for the size-density of the consumer coupled to an ordinary differential equation for the resource concentration. The second is called the DELAY formulation and employs a renewal equation for the population level birth rate of the consumer, coupled to a delay differential equation for the (history of the) resource concentration. With each of the two formulations we associate a constructively defined semigroup of nonlinear solution operators. The trouble with the PDE formulation is that the solution operators are NOT differentiable, precluding rigorous linearisation. (The underlying reason is the same as for state-dependent delay equations: we need to differentiate with respect to a quantity that appears as argument of a function that may not be differentiable.) The DELAY formulation does not suffer from this technical difficulty and the Principle of Linearized Stability holds [1]. The two semigroups are intertwined by a non-invertible operator. Under certain conditions this 'equivalence' yields a rather indirect proof of the Principle of Linearized Stability for the PDE formulation [2].
[1] Diekmann, O., & Gyllenberg, M. Equations with infinite delay: blending the abstract and the concrete. Journal of Differential Equations (2012) 252 : 819-851
[2] Barril, C., Calsina, À., Diekmann, O., & Farkas, J. Z. (2021). On the formulation of size-structured consumer resource models (with special attention for the principle of linearized stability). Mathematical Models and Methods in Applied Sciences (2022) 32 : 1141-1191
Title: Exact rate of acceleration in propagation determined by KPP equations with nonlocal diffusion and free boundaries.
Abstract: A new phenomenon in nonlocal diffusion models is that accelerated propagation may happen, that is, the propagation speed could be infinite, which never occurs in the corresponding local diffusion model with compactly supported initial data. In this talk we will look at such a phenomenon for the KPP equation with nonlocal diffusion and free boundaries. For several natural classes of kernel functions appearing in the nonlocal diffusion term, we will show how the exact rate of acceleration can be determined. The talk is based on joint work with Dr Wenjie Ni.
Title: Synchronization of piecewise-smooth networks
Abstract: We consider synchronization of networks of piecewise smooth oscillators. When dealing with solutions of piecewise smooth systems two main difficulties must be overcome: i) The fundamental matrix solution is not unique in general; ii) The large dimension of the problem requires efficient numerical techniques. In this talk we address both issues. We give a well-defined fundamental matrix solution along synchronous solutions and we use its structure to derive a suitable numerical technique for the computation of the Floquet multipliers of the whole network. This is a joint work with Luca Dieci.
Title: Dynamics of lattice models arising from neural networks
Abstract: A number of lattice dynamical systems modeling evolution of biological or artificial neural networks will be introduced. An emphasis will be given to the interconnection structures of the neural networks and how they affect the stability of the networks. Effects of uncertain external input and/or time delays in responding to activation will also be addressed. Mathematical tools and techniques used to study the dynamics of these networks will be presented.
Title: Data-driven Computation of Stochastic Dynamics
Abstract: Stochastic differential equations (SDEs) play an important role in many scientific applications. It is difficult to fully understand the long-term dynamics of SDEs using rigorous approaches. Conventional numerical methods also have certain limitations. In this talk, I will review some of my recent work on data-driven computation methods for computing long-term dynamics of stochastic differential equations. This includes the computation of the invariant probability measure, the quasi-stationary distribution (QSD), and the speed of convergence towards the invariant probability measure. If time permits, I will briefly introduce some applications in sensitivity analysis and deep learning theory.
Title: The averaging principle for SDEs with monotone coefficients
Abstract: Averaging principle is an effective method for investigating multi-scale dynamical systems. In this talk, we will discuss three types of averaging principle for monotone stochastic differential equations, which includes monotone SPDEs, stochastic complex Ginzburg-Landau equations and finite dimensional monotone SDEs. Furthermore, we investigate the small fluctuations of the monotone SDEs around its average, and show that the normalized difference weakly converges to an Ornstein-Uhlenbeck type process, which can be viewed as a functional central limit theorem. This talk is based on joint works with Mengyu Cheng and Michael Röckner.
Title: “Mild solutions” and variation of constants formula for delay differential equations
Abstract: The method and the formula of variation of constants for ordinary differential equations (ODEs) is a fundamental tool to analyze the dynamics of an ODE near an equilibrium. It is natural to expect that such a formula works for delay differential equations (DDEs), however, it is well-known that there is a conceptual difficulty in the formula for DDEs. In this talk, we discuss the variation of constants formula for DDEs by introducing the notion of a mild solution, which is a solution under an initial condition having a discontinuous history function. We will also apply the formula to show the principle of linearized stability and the Poincaré–Lyapunov theorem for DDEs, where we do not need to assume the uniqueness of a solution.
Title: Long transient dynamics in stochastic systems with applications
Abstract: Systems’ essential dynamics over finite time are referred to as transient dynamics. They are thought to be more relevant to physically observed dynamical behaviors. In this talk, we are mainly concerned about long transient dynamics in stochastic systems. In particular, we consider two different models with small noise perturbation arising from population dynamics, where species only coexist over a long finite time period and go to extinction in the long run. To capture such transient persistent dynamics, we use quasi-stationary distributions (QSDs) and study their noise-vanishing asymptotic. Since QSD is closely related to the spectrum of the Fokker-Planck operator, our method is mainly based on PDE analysis. It is worthwhile to mention that the second-order coefficients of the Fokker-Planck operator are degenerate on the boundary for any fixed noise and vanish in the zero-noise limit, resulting in essential technical difficulties. In the end of the talk, I would list some topics for future work.
Title: Analysis of long transient dynamics and detecting early warning signals of regime
shifts in a class of predator-prey models featuring two timescales.
Abstract: The leading role of long transient dynamics in ecological timescales can be very important
in explaining regime shifts. However, analytical techniques for studying long transients in relevant timescales in three or higher-dimensional ecological models is still at its infancy. In this talk, I will consider a three-dimensional predator-prey model featuring two-timescales that governs the interaction between two species of predators competing for their common prey with explicit interference competition. I will consider two different scenarios in a parameter regime near singular Hopf bifurcation of the coexistence equilibrium point. In one case, the system exhibits bistability between a periodic attractor and a boundary equilibrium state, with long transients characterized by rapid small-amplitude oscillations and slow variation in amplitudes, while in the other, the system exhibits chaotic mixed-mode oscillations, featuring concatenation of small and large-amplitude oscillations, as long transients before approaching a stable limit cycle. To analyze the transients, the system is reduced to a suitable normal form near the singular Hopf point. Exploiting the separation of timescales and the underlying geometry of the normal form, the transient dynamics are analyzed. The analyses are then used to devise methods for identifying early warning signals of an abrupt transition resulting in an extinction of one of the species or a large population transition leading to an outbreak. I will finally end with some preliminary analysis on extending the results to stochastic settings.
Title: Stochastic stability of invariant measures
Abstract: Dynamical systems are inevitably subject to noise perturbations, making the study of the stability of dynamical systems under noise perturbations a fundamental problem. Often used to characterize the complexity of dynamical behaviours, invariant measures, especially the physical ones, are believed to be stochastically stable. This is well-known for hyperbolic systems but remains widely open for more general systems. The main purpose of this talk is to present our recent results on this issue for conservative systems or systems having conservative quantities. A special attention will be paid to the “strong” stochastic stability of invariant measures and its potential applications.
Title: On determining the existence of periodic orbits
Abstract: We present theoretical settings of three approaches to computer-assisted proving the existence of periodic orbits of flows generated by ordinary differential equations. The first and the second methods are based on the notion a brick, i.e. an isolating block having the invariant part empty. If a (possibly) large isolating block is filled-in with bricks, some clusters of bricks are properly ordered, and suitable relations among the bricks hold (distinct for each one of these methods), then the flow has a periodic orbit. The reference to the first approach is [1], while the second one is motivated by the paper [4] and it will be given in [2]. In the third approach, based on the papers [3] and [5], the existence of a periodic orbit in a rotating flow is guaranteed, provided some conditions on singular homology cycles of a section of an index pair with respect to a time-discretization of the flow hold.
References:
[1] M.Mrozek, R.Srzednicki, J.Thorpe, T.Wanner, Combinatorial vs. classical dynamics: Recurrence, Comm. Nonlin. Sc. Numerical Simulation 108 (2022) 106226, 1-30.
[2] M.Mrozek, R.Srzednicki, J.Thorpe, T.Wanner, Combinatorial vs. classical dynamics: Recurrence: A Lefschetz fixed point theorem for periodic orbits, in preparation.
[3] M.Mrozek, R.Srzednicki, F.Weilandt, A topological approach to the algorithmic computation of the Conley index for Poincare Maps, SIAM J. Appl. Dynamical Systems 14 (2015), 1348-1386.
[4] R.Srzednicki, On periodic solutions inside isolating chains, J. Differential Equations 165 (2000), 42-60.
[5] R.Srzednicki, On determining the homological Conley index of Poincare maps in autonomous systems, Topol. Methods Nonlinear Anal. 60 (2022), 5-32.
Title: Can one hear the shape of high-dimensional landscape?
Abstract: Potential functions used in optimizations, dynamics applications, and machine learning etc. can be rather complicated in term of their structures and properties especially in very high dimensions. Due to lacking of knowledge on concrete forms of potential functions in real applications, even the determination of their basic structures and properties is a challenging problem in both mathematical analysis and numerical simulations. This talk presents a probabilistic approach to investigate the landscape of potential functions, including those in high dimensions, by using an appropriate coupling scheme to couple two copies of the overdamped Langevin dynamics of the potential functions. It can be theoretically shown that for potential functions with single or multiple wells, the coupling time distributions admit qualitatively distinct exponential tails in terms of noise magnitudes. In addition, a quantitative characterization of the non-convexity of a multi-well potential function can also be obtained via linear extrapolation. These theoretical findings thus suggest a promising approach to probe the shape of a potential landscape through the coupling time distributions at least numerically. Such a detection approach shares the same spirit with the well-known problem of “Can one hear the shape of a drum?” proposed by Kac in his famous 1966 paper. A Numerical example on loss functions of neural networks with different sizes will be demonstrated. This talk is based on a recent joint work with Yao Li at UMASS and Molei Tao at Georgia Tech.
Title: KAM Theorems in application for multi-scale systems
Abstract: My talk is about the quasi-periodic motions in multi-scaled Hamiltonian systems.
It consists of three parts. At first, I will introduce the KAM type results in nearly integrable Hamiltonian systems. In the second part, I will introduce the back ground and corresponding definitions of multi-scale nearly integrable Hamiltonian systems. After then, I will introduce that what is Poincare mechanics and certain interesting results corresponding to this problem. I will also simply introduce the technical ingredients of our work.The last part, I will talk about the applications of the persistence result to the nonlinear oscillator, which is our recent result. A joint with Prof. Y.Yi and Prof. Y.Li.
Workshop on Dynamics of Infectious Diseases
Title: Reclustering of populations based on mobility-driven well-mixing using reinforcement learning - disease spread insights
Abstract: In this talk we present an algorithm designed to recast a population (here that of the US) through the lens of county population's mobility patterns. The result is a reclustering of the US population in regions that are not geographically distinct, but instead have low inter-region travelling. Such subpopulations are now well-mixed from the perspective of an ODE model of SEIR-type, hence in-depth analyses of infection spread are much better supported. We highlight differences and similarities in the epidemic evolution of Covid-19 in 2020 in the US, following the population reclustering, and the interplay between population density and sizes of the clusters, differences between regional incidence (peaks) and sizes of reported incidence state-wide Mar-July of 2020, and their implications on localized NPI measures. Further, we look at ways to use the current Covid-19 based modelling to derive insights on the impact of regionality/clustering for different regions of the world. Joint work with: D. Lyver, M. Nica & E. Thommes (U. of Guelph) and C. Cot and G. Cacciapaglia (INRS & Univ. of Lyon).
Title: Analysis of metapopulation models of the transmission of SARS-CoV-2 in the United States
Abstract: During the COVID-19 pandemic, renewal equation estimates of time-varying effective reproduction numbers were useful to policymakers in evaluating the need for and impact of mitigation measures. Our model is stratified by age, 0-4, 5-9, … 75+ years, and location, the 50 states plus District of Columbia. Expressions from such heterogeneous host population models include subpopulation reproduction numbers, contributions from the above-mentioned infectious states, metapopulation numbers, subpopulation contributions, and equilibrium prevalence. The model can be used to identify optimal allocations of limited resources among subpopulations. We illustrate the utility of such analytical results by simulating two hypothetical vaccination scenarios which are compared with the actual program estimated from one of the CDC’s nationwide seroprevalence surveys conducted from mid-summer 2020 through the end of 2021.
Title: Modelling Immunity
Abstract: Immunity is gained from infection and vaccination, and it can decay over time. Immunity is also an individual and a population-level characteristic. We will discuss mathematical models of immunity in-host and in populations. The models are used to track immunity dynamics and determine the feasibility of control strategies that enhance immunity and minimize infection.
Title: Spatial dynamics of a pest population with stage-structure and control
Abstract: We study an integro-difference model for a pest population with stage structure and control on each stage. When the spatial domain is infinite, we establish the spreading speeds and existence of traveling waves; when the spatial domain is finite, we first establish threshold conditions in terms of an eigenvalue of an associated eigenvalue problem to determine population persistence and extinction, and then define the net reproductive rate and develop equivalent threshold conditions for persistence and extinction by using the net reproductive rate. The cases where the reproduction function is monotone and nonmonotone are both investigated.
Title: Existence and uniqueness of solutions of nonlinear Cauchy type problems for first order
fractional differential equations
Abstract: In this presentation, I shall introduce definitions of the first order and higher order fractional derivatives. Then I shall present the equivalences between the first-order fractional differential equations
and integral equations, and the existence of solutions of the initial value problems for first-order fractional differential equations.
Title: Re-thinking the Mathematical Theory of Epidemics
Abstract: Modeling of epidemics of infectious diseases using differential equations has a long history and many applications to various diseases, most recently to the COVID-19 pandemic. It is through the wide application of mathematical modeling during the COVID-19 pandemics, we realized that standard differential equations models, simple or complex, have a tendency to significantly over-predict (or over-project) the size of an epidemic. In searching for reasons for this ``annoying’’ tendency, many questions can be asked, one of them is: should mathematical models be validated before projection are made?
The traditional belief is that mathematical models of epidemics are built based on the best science of mechanisms of disease transmission and spread, and should be trusted. The resulting dynamical system would truthfully predict the disease transmission dynamics, after the calibration using disease data. However, if the disease data used for calibration are positive case reports, which is a proportion of the true number of infections, then we are using a deterministic model with information from partial observations of the state variables. As it turns out (I will show in this talk), when only partial observations are available for model calibration, our mathematical model can behave very badly, and model validation becomes crucial before reliable predictions can be made.
Title: West Nile virus diffusive models with climate warming and seasonal succession
Abstract: This talk deals with mathematical models describing the diffusive dynamic of West Nile virus (WNV). For the spatially-independent WNv model, the usual basic reproduction number $R_0$ is given and for the diffusive WNv model in a bounded domain, the basic reproduction numbers $R_0^N, R_0^D$ are defined. To model and explore the expanding front of the infective region, a reaction-diffusion problem with free boundaries is proposed. The spatial-temporal risk index $R_0^F(t)$, which involves regional characteristic and time, is defined. Sufficient conditions for the virus to vanish or spread are given. Our results suggest that the spreading or vanishing of the virus depends on the initial number of infected individuals, the area of the infected region, the diffusion rate, and other factors. Moreover, we establish new WNv models to describe the impart of climate warming and seasonal succession.
Title: Hybrid Formation Control of Multi-Agent Systems with Time Delays
Abstract: As one of the most significant issues in the distributed coordination of multi-agent systems, formation control has received increased attention in recent years due to its wide applications in satellite formation flying, exploration, surveillance and rescue. The formation control problem aims to design suitable protocols such that a group of agents can reach a desired geometric structure from arbitrary initial positions. This talk discusses the leader-following formation problem of the unmanned aerial vehicle (UAV) swarm by integrating hybrid event-triggered control and pinning mechanism, in order to efficiently reduce control resources consumption and transmission redundancy. Meanwhile, delayed control inputs are implemented for more practical applications and comparisons are made with real-time control; topology switching and strong dynamics nonlinearity are also taken into account. In addition, gyroscopic force and braking force are utilized for maintaining collision-free movements. Sufficient robust formation criteria are derived to guarantee convergence of the corresponding formation error dynamics without exhibiting Zeno behavior. Examples and numerical simulations are provided to illustrate the effectiveness of the proposed approach.
Title: The Dynamics and Transmission of COVID-19 epidemic in Pakistan using SEIR model and its future prediction
Abstract: The aim of the present research is to model transmission of COVID-19 epidemic disease. In order to see the behavior of these infectious diseases and its future prediction we have considered the real data from WHO. The aim of the present study is to considered COVID-19 epidemic model. The total papulation is divided in four sub classes as susceptible S, exposed E, infected I and recovered class R. The infectious diseases COVID-19 is formulated using SEIR model. The real data is taken from Who and we have calculated basic reproduction number and found that numerical value is R0=2.03 which shows that the diseases is spreading within the papulation. Furthermore, stability analysis of the model at disease free equilibrium DFE and endemic equilibriums EE is performed to observe the dynamics and transmission of the model. Finally, the AB fractional model is solved numerically. To show the effect of the various embedded parameters like fractional parameter α on the model, various graphs are plotted. It is worth noting that the on base of our investigation, we have predicted the spread of disease for next 200 days.
Title: An epidemic model for reinfection dynamics with heterogeneous susceptibility
Abstract: Individuals in a population vary in susceptibility. To explore the impact of the distributed susceptibility, we consider an epidemic model, where individuals acquire a degree of susceptibility with a probability after infection. It is shown that heterogeneous susceptibility adds complexity to the reinfection dynamics, and changes in the distribution of susceptibility may cause unexpected epidemics. We revisit the epidemic model studied by Katriel in 2010. The study is partly a joint work with Ryosuke Omori.
Title: Age-structured population dynamics with nonlocal diffusion
Abstract: Individuals need to be mature enough to disperse and recent observations demonstrate that nonlocal diffusion processes are more applicable to many biological and epidemiological problems compared with random diffusion processes. Consequently, it is more reasonable to study age-structured population models with nonlocal (convolution) diffusion. In this talk, I will introduce some recent results on the basic theory for age-structured population dynamics with nonlocal diffusion (Based on joint studies with Hao Kang).
Title: Complex dynamics of epidemic models on adaptive networks
Abstract: There has been a substantial amount of well-mixing epidemic models devoted to characterizing the observed complex phenomena (such as bistability, hysteresis, oscillations, etc.) during the transmission of many infectious diseases. A comprehensive explanation of these phenomena by epidemic models on complex networks is still lacking. In this talk, we study epidemic dynamics in an adaptive network model proposed by Gross et al, where the susceptibles are able to avoid contact with the infected by rewiring their network connections. Such rewiring of the local connections changes the topology of the network and inevitably has a profound effect on the transmission of infectious diseases, which in turn influences the rewiring process. We rigorously prove that such an adaptive epidemic network model exhibits degenerate Hopf bifurcation, homoclinic bifurcation, and Bogdanov-Takens bifurcation. Our study shows that human adaptive behaviors to the emergence of an epidemic may induce complex dynamics of disease transmission, including bistability, and transient and sustained oscillations, which contrast sharply with the dynamics of classic network models. Our results yield deeper insights into the interplay between the topology of networks and the dynamics of disease transmission on networks.
Title: Predictive modelling and forecasting of the mosquito abundance and risk of West Nile virus
Abstract: The transmission and spread of mosquito-borne disease such as West Nile virus (WNV) in north America are weather-sensitive. Weather conditions, such as daily average temperature and precipitation, not only affect the abundance but also the biting behavior of the vector mosquitoes, thus determining the outbreak and spread of WNV. In this talk, I will present a hybrid model for weekly forecasting of the abundance and risk of infection of WNV, integrating a weather-driven statistical model and a compartmental model mimicking the transmission of WNV among mosquitoes, birds, and humans. The predictive models are evaluated using the data of human infection, mosquito surveillance and viral test in Peel Region, Ontario, Canada. With the weekly mosquito surveillance data and the weather forecast, our real time predictive modelling allows us to forecast the weekly vector abundance and risk of WNV infection which has important public health implications. I will conclude with a summary of preliminary dynamics of such hybrid models with weather conditions. This is a joint work with Huaiping Zhu and Nick Ogden in collaboration with Peel public Health.
Title: Bifurcation in a diffusive predator-prey model with discontinuous harvesting
Abstract: In this presentation, we explore the potential bifurcations in a diffusive predator-prey model incorporating discontinuous harvesting. Specifically, we delve into the existence of sliding mode and examine various types of sliding mode bifurcations, including boundary node, grazing, buckling, and crossing bifurcations. To illustrate our findings, we utilize numerical simulations, which showcase how the system can develop diverse spatial patterns based on factors such as the harvesting threshold, cost of fear, and initial conditions. This is a joint work with Drs. Zhao and Zhang.
Title: The Linear Stability and Basic Reproduction Numbers for Autonomous FDEs
Abstract: The basic reproduction number R0 is an important concept in population biology. As a threshold quantity for population dynamics, it is unquestionably one of the most valuable mathematical ideas brought to theoretical ecology and epidemiology. In this talk, I will report our recent research on the linear stability and R0 for autonomous and cooperative functional differential equations (FDEs). In particular, we will give a general formula of R0 for autonomous and compartmental FDE models, where both the production (infection) and internal transition have time delays. We also extend this theory to abstract autonomous FDEs on an ordered Banach space so that it can be applied to time-delayed reaction-diffusion systems. As an illustrative example, we establish the threshold dynamics for a time-delayed population model of black-legged ticks in terms of R0.
Workshop on Dynamics and Evolutionary Equations
– in honor of Geneviève Raugel (1951 - 2019)
Organizer: Romain Joly and Yingfei Yi

As a part of the 4th International Conference on Dynamics of Differential Equations - in memory of Jack K. Hale, this special workshop is in honor of Professor Geneviève Raugel.
Professor Raugel obtained her Ph.D degree from Uniersité Rennes in 1978. Her career had been associated with CNRS, first as a researcher (1978-1994) then a research director (1994-2019). In particular, she worked at the Orsay Math Lab of CNRS affiliated to the University of Paris-Sud since 1989. Her early research interest lied in numerical analysis concerning finite element discretization of PDEs and related bifurcation problems. Since the mid of 1980s, she started working on infinite dimensional dynamical systems especially on the dynamics of evolution equations including reaction-diffusion equations, damped wave equations, and Navier-Stokes equations. Widely regarded as a top researcher and a world-leading expert in these areas, she was especially recognized by her fundamental contributions to the subjects of global attractor and disturbances, thin domain problems, and infinite dimensional Morse-Smale structures.
Professor Raugel has made many distinguished services to the international mathematical community. In particular, she was a co-founder of this conference series, co-chaired its executive committee, and organized the 2nd conference of the series in Luminy, France in 2016.
Title: Universal dynamics of invasion fronts
Abstract: Front propagation into unstable states plays an important role in organizing structure formation in many spatially extended systems. When a trivial background state is pointwise unstable, localized perturbations typically grow and spread with a selected speed, leaving behind a selected state in their wake. A fundamental question of great interest is to predict the propagation speed and the state selected in the wake. The marginal stability conjecture postulates that speeds can be universally predicted via a marginal spectral stability criterion. In this talk, we will present background on the marginal stability conjecture and present some ideas of our recent conceptual proof of the conjecture in a model-independent framework focusing on systems of parabolic equations.
Title: Semi-discrete optimal transport problems: finding the network structure
by exploiting star shapedeness and use of Newton's method
Abstract: In this talk, we propose a novel implementation of Newton's method for solving semi-discrete optimal transport problems for cost functions which is a positive combination of $p$-norms, $1<p<\infty$. It is well understood that the problem reduces to finding a subdivision of a bounded region in Laguerre cells, and we prove that the Laguerre cells are star-shaped with respect to the target points. By exploiting the geometry of the Laguerre cells, we obtain an efficient and reliable implementation of Newton's method to find the sought network structure. We give some implementation details and numerical results. Joint work with Daniyar Omarov, Georgia Tech.
Title: A global bifurcation diagram for a one-parameter family of nonautonomous scalar ODE's driven by a minimal flow.
Abstract: The description of nonautonomous bifurcation patterns is of growing interest in the scientific community, both for its theoretical interest and for its possible applications to the analysis of mathematical models. In the talk, in the wake of [1] and using results and methods of [2], conditions on the coefficients of the one-parameter family $x'(t)=\varepsilon(a(t)+b(t)\,x (t)) + c(t)\,x^2 (t) -x^3 (t)$ as $\varepsilon$ varies are established to describe the global diagram of the motion. Such conditions include the recurrency of the coefficients. Joint work with Carmen Nunez.
[1] R. Fabbri, R. Johnson, F. Mantellini, "A nonautonomous saddle-node bifurcation pattern", Stochastics and Dynamics, Vol. 4, No. 4 (2004) 335-350.
[2] J. Duenas, C. Nunez, R. Obaya, "Bifurcation theory of attractors and minimal sets in d-concave nonautonomous scalar ordinary differential equations", Journal of Differential Equations, 361 (2023) 138-182.
Title: Computing the Global Dynamics of Parameterized Systems of ODEs
Abstract: We present a combinatorial topological method to compute the dynamics of a parameterized family of ODEs. A discretization of the state space of the systems is used to construct a combinatorial representation from which recurrent versus non-recurrent dynamics is extracted. Algebraic topology is then used to validate and characterize the dynamics of the system. We will discuss the combinatorial description and the algebraic topological computations and will present applications to systems of ODEs arising from gene regulatory networks.
Title: Combinatorial structure of continuous dynamics in gene regulatory networks
Abstract: We first describe the mathematical foundation of DSGRN (Dynamic Signatures Generated by Regulatory Networks), an approach that provides a combinatorial description of global dynamics of a network over its entire parameter space. Finite description allows comparison of parameterized dynamics between hundreds of networks to discard networks that are not compatible with experimental data. We also describe a close connection of DSGRN to Boolean network models that allows us to view DSGRN as a platform for bifurcation theory of Boolean maps. We discuss several applications of this methodology to systems biology.
Title: Stochastic Speed and Shape Corrections for Travelling Patterns in Random Media
Abstract: We describe a framework to uncover the shape and speed corrections caused by adding noise-terms to PDEs that support travelling wave solutions. We consider both parabolic and dispersive systems, and noise terms that can be Lipschitz or non-Lipschitz. By using perturbative techniques, we avoid the need to use the comparison principle. In some cases, it is no longer sufficient to use the standard linear operators associated to the deterministic travelling waves, which uncovers a surprising connection with singular perturbation theory. Joint work with C. Hamster and R. Westdorp.
Title: Linearized instability for differential equations with dependence on the past derivative
Abstract: We provide a criterion for instability of equilibria of equations in the form $\dot x(t) = g(x_t', x_t)$, which includes neutral delay equations with state-dependent delay. The criterion is based on a lower bound $\Delta >0$ for the delay in the neutral terms, on regularity assumptions of the functions in the equation, and on spectral assumptions for an approximating semigroup. Estimates in the $C^1$-norm, a manifold containing the state space $X_2$ of the equation, another manifold contained in $X_2$, and an invariant cone method are used for the proof. A mechanical example illustrates possible applications. (Joint work with Jaqueline Godoy Mesquita).
Title: Disturbing the Big Bang
Abstract: We will give an introductory talk on the dynamics of the Big Bang singularity and perturbations thereof. This topic has attracted a great deal of attention of both mathematicians and physicists since the heuristic approach of Belinski–Khalatnikov–Lifshitz (known as BKL conjecture/picture) and the Mixmaster attractor construction of Misner. We will see how a specific perturbation of the Big Bang singularity will unravel well-known and brand-new dynamical features. Moreover, we will see how such perturbations yield good (or bad) approximating schemes of the usual Einstein's general theory of relativity. These results were fruit of collaborations with K.E. Church (U Montreal), V.H. Daniel (Columbia U), J. Hell (FU Berlin), O. Hénot (McGill U), J.P. Lessard (McGill U), H. Sprink (FU Berlin) and C. Uggla (Karlstad U).
Title: Permanent charge effects on ionic flows
Abstract: Permanent charge (mathematical model for protein structure) is the most important structure of an ion channel and is the major controller for properties of ionic flows through the ion channel. Another important factor for ionic flow specifics is the boundary conditions. In this talk, we will report our studies toward an understanding of effects of interplays between permanent charge and boundary conditions on ionic flow via a quasi-one-dimensional Poisson-Nernst-Planck (PNP) model.
Permanent charges considered here are restricted to a special case of piecewise constant with one non-zero portion. For ionic mixtures with one cation (positively charged) species and one anion (negatively charged) species, a fairly rich behavior of permanent charge effects has been revealed from rigorous analyses based on a geometric framework for PNP and from numerical simulations guided by the analytical results. For ionic mixtures with three ion species: two cations and one anion, richer behavior is expected and our preliminary analysis and case studies identify, in concrete manner, a number of these, including some surprising ones.
TITLE: Critical transitions in nonautonomous ordinary equations with concave derivative
ABSTRACT: A function with finite asymptotic limits gives rise to a transition equation between a "past system" and a "future system". We analyze this situation in the cases of nonautonomous coercive scalar ODEs with concave derivative with respect to the state variable, assuming the existence of three hyperbolic solutions for the limit systems, in which case the upper and lower ones are attractive. The different global dynamical possibilities are described in terms of the internal dynamics of the pullback attractor: cases of tracking of the two hyperbolic attractive solutions or lack of it (tipping) arise. This analysis allows us to present cases of rate-induced critical transitions, as well as cases of phase-induced and size-induced tipping. Our conclusions are applied in models of mathematical biology and population dynamics: we describe rate-induced tracking phenomena causing extinction of a native species or invasion of a non-native one. This is a joint work with Jesús Dueñas and Rafael Obaya.
Title: Analysis and modeling of the modular Burgers equation
Abstract: I will review the state of art in analysis and modeling of the Burgers’ equation with a modular advection term. The modular Burgers’ equation admits a traveling viscous shock with a single interface, which is stable against smooth and exponentially localized perturbations. In contrast, it can be shown with the help of energy estimates and numerical simulations that the evolution of shock waves with multiple interfaces leads to finite-time coalescence of two consecutive interfaces. A precise scaling law of the finite-time extinction is supported by the interface equations and by numerical simulations
Title: Selection criteria for invasion fronts
Abstract: I will overview recent efforts that strive to characterize both the speed of invasion fronts and the selected states in the wake of invasion, from a conceptual, somewhat model independent point of view. The first part of the talk will be concerned with pulled and pushed fronts and the marginal stability conjecture, focusing on analytical and computational tools for determining wavespeeds. In the second part, I will present some intriguing examples of invasion mechanisms that are based on higher spatio-temporal resonances. This is joint work with Montie Avery and Matt Holzer.
Title: Projection Techniques and Reduced Order Modeling for Data Assimilation
Abstract: Data assimilation (DA) techniques combine physical model forecasts with data or observations to predict future model states typically with some measure of uncertainty. In Bayesian DA this is done by expressing the posterior as proportional to the likelihood times the prior. Two common classes of techniques are Kalman Filter (KF) based techniques that parameterize the uncertainty in the posterior distribution as a Gaussian and Particle Filter (PF) based techniques that express posterior distributions in terms of weighted particles (physical model states) but generally suffer from curse of dimensionality issues. Motivated by computational dynamical systems techniques we develop techniques initially based on the use of reduced order physical models but more recently emphasizing the use of reduced order observational or data models. We illustrate the effectiveness of the techniques developed on both fast and slow systems, in particular versions of the Lorenz ’96 model and discretized 2D shallow water equation.
Title: On solution manifolds
Title: Response solutions in degenerate, quasi-periodically forced nonlinear oscillators
Abstract. For a quasi-periodically forced differential equation, response solutions are quasi-periodic ones whose frequency vector coincides with that of the forcing function and they are known to play a fundamental role in the harmonic and synchronizing behaviors of quasi-periodically forced oscillators. These solutions are well-understood in quasi-periodically perturbed nonlinear oscillators either in the presence of large damping or in the non-degenerate cases with small or free damping. This talk will present some recent results on the existence of responsive solutions in degenerate, quasi-periodically forced nonlinear oscillators with small or free damping.
Title: Modelling the impact of human precaution on disease dynamics and its evolution
Abstract: In the past three years, we have witnessed or seen or felt that the human (host) behaviour/precaution during an epidemic/pandemic can play a significant role in controlling the disease dynamics. In this talk, we discuss, by mathematical models, the impact of human precaution level on disease dynamics as well as the evolution of the precaution level.
Organizing Committee
- Luca Dieci - Georgia Institute of Technology
- Hans-Otto Walther - Universitaet Giessen
- Huaiping Zhu - York University (Chair)
Scientific Committee
- Jose Maria Arrieta - Complutense University of Madrid
- Alexandre Carvalho - University of São Paulo
- Giorgio Fusco - Università degli studi dell'Aquila
- Romain Joly - Université Grenoble-Alpes
- Carlos Rocha - University of Lisbon
- Kunimochi Sakamoto - Hiroshima University
- Sjoerd Verduyn Lunel - Utrecht University
- Huaiping Zhu - York University
- Yingfei Yi - University of Alberta (Chair)
Canadian local coordinating Committee
- Jacques Bélair - Université de Montréal
- Sue Ann Campbell - University of Waterloo
- Michael Li - University of Alberta
- Yingfei Yi - University of Alberta
- Xiaoqiang Zhao - Memorial University
- Huaiping Zhu - York University (Chair)