Topics

• "For it is unworthy of excellent men to lose hours like slaves in the labour of calculation which would safely be relegated to anyone else if machines were used."

  This statement, uttered by Gottfried Wilhelm Von Leibniz in 1685, could well be taken as one of the manifestos of modern computing, and the seventeenth century witnesses a true outburst of inventions in this area, powered by pivotal mathematical research.
  Note :  Before we continue, notice that we seem to have skipped the Renaissance. The Renaissance is usually considered to have started in Italy, specifically in Florence, as early as the end of the thirteenth century. By the fifteenth century all of Italy was experiencing this rebirth, but it was only in the sixteenth century that the rest of Europe can be considered to have entered the Renaissance in full. Chronologically at least, and for the purposes of this course, we will use the term Middle Ages to include at least the early Renaissance. After all, the (Late) Middle Ages overlap to a considerable degree with the Renaissance, and the latter had different timelines in different parts of Europe,
• Here is a brief timeline spanning the period considered in this and the following lecture:
  • 1612-1614:  John Napier uses the printed decimal point, devises logarithms, and uses numbered sticks, or Napier's Bones, for calculating
  • 1614:  John Napier publishes his Cannon of Logarithms
  • 1617:  John Napier publishes Rabdologia describing Napier's bones and Multipicationis Promptuarium
  • ca 1620:  Use of sector gaining popularity in Europe
  • 1620:  Robert Napier publishes John Napier's work Constructio
  • 1622:  William Oughtred invents the circular slide rule on the basis of Napier's logarithms
  • 1623:  Wilhelm Schickard designs a calculating clock with a gear-driven carry mechanism to aid in multiplication of multi-digit numbers. It is believed to be the first true mechanical calculating machine
  • 1623:  The Works of that Famous Mathematician Mr. Edmund Gunter published
  • 1624:  Henry Briggs publishes the first set of modern logarithms
  • 1628:  Adrian Vlacq publishes the first complete set of modern logarithms
  • 1642-1643:  Blaise Pascal creates a gear-driven adding machine called the Pascalene, the first mechanical adding machine
  • ca 1650:  Sliding stick form of the slide rule developed
  • 1666:  In England, Samuel Morland produces a mechanical calculator that can add and subtract
  • 1666:  Gaspard Schott publishes Organum Mathematicum
  • 1672:  Samuel Morland publishes The Description and Use of Two Arithmetic Instruments
  • 1673:  Rene Grillet describes the general form of his adding machine
  • 1674:  Gottfried Leibniz builds the Stepped Reckoner, a calculator using a stepped cylinder gear
• The first instruments I want to consider here are the quadrant and the sector (see Michael Williams, A History of Computing Technology. IEEE Computer Society Press, 1997, pp 66 - 83). I will mention here Edmund Gunter (1581 - 1626):

  "He made a mechanical device, Gunter's scale, to multiply numbers based on the logs using a single scale and a pair of dividers. It was called the gunter by seamen and was an important step in the development of the slide rule. Gunter published his description in 1624 in Description and Use of the Sector, the Crosse-staffe and other Instruments."

   

  

  Closeup on the Gunter Scale
  (From The Museum of HP Calculators)

   

  

  Simple Gunter Quadrant
  (from National Maritime Museum, Greenwich, England)

   

  The quadrant (see also the illustration in Lecture 7) derives from the astrolabe, "which Gunter folded into four parts and compressed so that they would fit on a quarter circle." [ from Williams, op. cit. ]. Although Gunter was not the inventor of the quadrant, he contributed a crucial improvement: for the first time, some of the scales engraved on the instrument are logarithmic, as in his scale.

   

  

  Ptolemy observing the stars with a quadrant, guided by Astronomy
  From the Whipple Library, Cambridge University

   

• The logarithms are the fundamental invention of John Napier (1550 - 1617). Before proceding any further, I will briefly review the definition and the elementary properties of logarithms.
  Consider the simple expression 10³ = 1000. We will say that 3 is the logarithm, in base 10, of 1000. Likewise, since 5² = 25, we will say that 2 is the logarithm, in base 5, of 25, and because 2⁶ = 64, that 6 is the logarithm, in base 2, of 64. If you like some generality, b^c = n can be restated by saying that c is the logarithm, in base b, of n or, more compactly, c = log _b (n).
  Some of the crucial properties of logarithms stem from the properties of exponentials:
  Since
  • b^m+n = b^mbⁿ
  • b^m-n = b^m/bⁿ
  • b^mn = (b^m)ⁿ
  • b⁰ = 1
  we have
  • log _b (xy) = log _b (x) + log _b (y)
  • log _b (x/y) = log _b (x) - log _b (y)
  • log _b (x^y) = y log _b (x)
  • log _b (1) = 0
  In plain words, logarithms allow multiplications to be reduced to additions, divisions to subtractions, and exponentiations to (simpler) multiplications or divisions. Nowadays we use mainly two types of logarithms, i.e. two bases: 10 and e = 2.71828… (see The Number e ) Notice also that logarithms provide a solution to the problem of physically representing large numbers. For example, in base 10, the logarithm of the number 1,000,000,000,000,000 is simply 15.
  Napier describes and discusses logarithms in his book Mirifici Logarithmorum Canonis Descriptio (1614). As the title suggests, the book is written in Latin. However, only two years later, an English translation appeared, to show once again how important scholarly discoveries would quickly become more generally available.

  "Seeing there is nothing (right well-beloved Students of the Mathematics) that is so troublesome to mathematical practice, nor that doth more molest and hinder calculators, than the multiplications, divisions, square and cubical extractions of great numbers, which besides the tedious expense of time are for the most part subject to many slippery errors, I began therefore to consider in my mind by what certain and ready art I might remove those hindrances. And having thought upon many things to this purpose, I found at length some excellent brief rules to be treated of (perhaps) hereafter. But amongst all, none more profitable than this which together with the hard and tedious multiplications, divisions, and extractions of roots, doth also cast away from the work itself even the very numbers themselves that are to be multiplied, divided and resolved into roots, and putteth other numbers in their place which perform as much as they can do, only by addition and subtraction, division by two or division by three."
  [ from John Napier ]

  It is interesting to note that my very brief introduction to the basics of logarithms is not the approach that Napier took.

  "Napier did not think of logarithms in an algebraic way, in fact algebra was not well enough developed in Napier's time to make this a realistic approach. Rather he thought by dynamical analogy. Consider two lines, AB of fixed length and A'X of infinite length. Points C and C' begin moving simultaneously to the right, starting at A and A' respectively with the same initial velocity; C' moves with uniform velocity and C with a velocity which is equal to the distance CB. Napier defined A'C' (= y) as the logarithm of BC (= x), that is

                                                   x
  A ————|———————————————— B
                     C →

         y
  A' ——|——————————————————… X
             C' →

  y = Nap.log x "
  

  Napier's logarithms, as initially constructed, were rather difficult to use, and lacked a fundamental property, log _b 1 = 0. Napier then proceeded to build mechanical devices that would somewhat automate arithmetical operations.

  "In his Rabdologiae, published in 1617. he described a method of multiplication using numbering rods with numbers marked off on them. The reason for publishing the work is given by Napier in the dedication, where he says that so many of his friends, to whom he had shown the numbering rods, were so pleased with them that they were already becoming widely used, even beginning to be used in foreign countries.

  Napier's numbering rods were made of ivory, so that they looked like bones which explains why they are now known as Napier's bones. To multiply numbers the bones were placed side by side and the appropriate products read off."

  A good reference for Napier's bones is Undusting Napier's Bones. A very interesting article on Napier's bones, and specifically on his proposal for a mechanical binary calculator, appeared on the Kuro5hin emagazine. It is entitled Napier's Chessboard Calculator.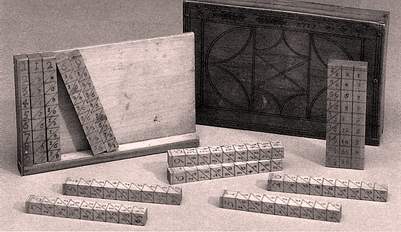

   

  

  Napier's Bones (ca 1717)
  ( From J A N Lee, Introduction to Computer Science )

   

  We can certainly agree that

  "Napier will be remembered for making one of the most important contributions to the advance of knowledge. It was through the use of logarithms that Kepler was able to reduce his observations and make his breakthrough which then in turn underpinned Newton's theory of gravitation. In the preface to the Mirifici Logarithmorum Canonis Descriptio, quoted above, Napier says he hoped that his logarithms will save calculators much time and free them from the slippery errors of calculations. Laplace, 200 year later, agreed, saying that logarithms:

  …by shortening the labours, doubled the life of the astronomer "

  [ from John Napier ]

• As we have noted, Napier's logarithms were somewhat unwieldy, also because of certain mathematical flaws (e.g. they wouldn't yield log _b (1) = 0). The situation improved when another famous character, Henry Briggs (1561 - 1630), entered the story. Briggs, in addition to his considerable mathematical ingenuity, was what we would call today a public relatuions man, and it was thanks to him that the scientific community ended up accepting logarithms as one of the tools of choice. Briggs, in discussions with Napier, proposed a more practical definition of logarithms, much more similar to the ine we use today. The base was to be 10, and now the relation log ₁₀ (1) = 0 would hold.

  "Briggs's mathematical treatise Arithmetica Logarithmica was published in 1624. This gave the logarithms of the natural numbers from 1 to 20,000 and 90,000 to 100,000 computed to 14 decimal places. It also gave tables of natural sine functions to 15 decimal places, and the tan and sec functions to 10 decimal places. In this book Briggs suggested that the logs of the missing numbers might be computed by a team of people and he even offered to supply specially designed paper for the purpose.

  The completed tables were printed at Gouda, in the Netherlands, in 1628 in an edition by Vlacq in which Vlacq had added the logarithms of the natural numbers from 20,000 to 90,000. The tables were also published in London in 1633 under the title of Trigonometria Britannica. The printing of the London edition took place after Briggs had died but he had asked his friend Henry Gellibrand to look after the project on his behalf. Gellibrand was professor of astronomy at Gresham College and was particularly interested in applications of logarithms to trigonometry. He therefore added a preface of his own on applications of logarithms to both plane trigonometry and to spherical trigonometry."

  Here is Williams' assessment of the importance of logarithms (op.cit., p. 105)

  "Logarithms were used extensively in all trades and professions that required calculations to be done. It is hard to imagine an invention which has helped the process of computation more dramatically that has logarithms., the one exception being the modern digital computer. During a conference held in 1914 to celebrate the 300th anniversary of the publication of the Descriptio it was estimated that, of all the calculations done in the previous 300 years, the vast majority had been done with the aid of logarithms."

• The next chapter in this story starts with Edmund Gunter, who saw the possibility of automating some of the operations with logarithms (e.g., the addition of two logarithms as equivalent to the multipication of two natural numbers) "by engraving a scale of logarithms on a piece of wood and then using a pair of dividers to add together the two values… Gunter's piece of wood soon became known as Gunter's Line of Numbers… Its use spread rapidly through England and was quickly popularized on the Continent…" [ op.cit., p. 106 ] Notice that the Gunter Scale was probably the first device to employ logarithms. It was not a slide rule yet, since there were no sliding parts.
  Gunter's work was picked up by William Oughtred (1574 - 1660).

  "Oughtred is best known for his invention of an early form of the slide rule. Edmund Gunter (1620) plotted a logarithmic scale along a single straight two foot long ruler. He added and subtracted lengths by using a pair of dividers, operations that were equivalent to multiplying and dividing. In 1630 Oughtred invented a circular slide rule. In 1632 he used two Gunter rulers so that he could do away with the dividers. He published Circles of Proportion and The Horizontal Instrument in 1632 describing slide rules and sundials."

  Oughtred's slide rule was originally circular

   

  

  A Charpentier Circular Slide Rule
  (The Oughtred Society)

   

  but straight rules were soon developed. Here is "the earliest known dated straight slide rule" at The Science Museum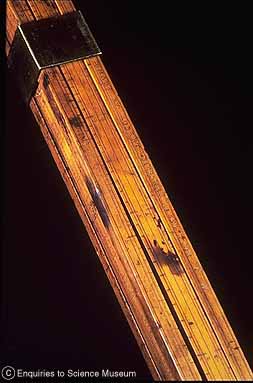

   

  

  Slide Rule by Robert Bissaker (1654)

   

• Strangely enough, the slide rule, in its innumerable incarnations dedicated to a huge reange of specific applications, had a slow beginning. Slide rules began to be used in earnest by engineers, accountants, and scientists only towards the end of the nineteenth century, and continued to be the tool of choice of such people until they were displaced by the electronic pocket calculator. Note that the Panama Canal was designed with the slide rule.

 
Readings, Resources and Questions

• Read Eric Marcotte's Introduction to the Slide Rule.  Other interesting places to visit are The Slide Rule section of the System Source Computer Museum, where a Java applet allows you to experiment with the various operations of a modern example, and Slide Rules and Other Mathematical Instruments.
• Browse through Florian Cajori's History of the Logarithmic Slide Rule. This is a classic study, published in 1909. Here are his conclusions concerning the invention of the slide rule:

  "Edmund Gunter invented a logarithmic line called Gunter's line, but not the slide rule; the straight edge slide rule was first invented by Edmund Wingate and explained by him in several publications, the earliest of which appeared in 1630. Such a slide rule was also given to the world in 1632 by William Oughtred, in a work prepared for the press by William Forster. Oughtred was the first to design a circular slide rule."

• Many of the instruments we have reviewed in this lecture are related to each other, often in interesting ways. Discuss some examples.